
The Astrophysics Spectator
The planets Jupiter and Saturn are very similar in their composition to the stars. What makes the stars and these planets different is the source of pressure that keeps each from collapsing under its own gravity. The source of pressure determines the size of a star or a planet. Within stars like the Sun, the pressure lasts for a finite time, but in the planets, the pressure is unvarying forever.
The stars are bodies of gas that generate heat through thermonuclear fusion; the heat provides the pressure that holds a star up. Within the main-sequence stars, which are stars burning hydrogen at their cores, hydrogen provides the heat, and within the more-complex red giants, helium at the star's core and hydrogen in a surrounding shell provide the heat. Remnant stars?degenerate (white) dwarfs and neutron stars?have exhausted their thermonuclear fuel; they are held up by a pressure arising from quantum mechanics; in the degenerate dwarfs, the pressure is provided by electrons, and in neutron stars, it is provided by neutrons and protons. The brown dwarfs are bodies that are too light to burn hydrogen. They are similar to the giant gaseous planets in their characteristics. Both they and the giant gaseous planets resemble the degenerate dwarfs in their source of internal pressure. Finally, the planets like Earth are held up by the pressure that atoms exert upon one-another.
The different sources of pressure are evident in the diagram below that shows the radius of an object as a function of mass. The planets, brown dwarfs, and degenerate dwarfs lie in the brown strip cutting horizontally across the diagram; the planets are at the low-mass end of the strip, the brown dwarfs are in the middle, and the degenerate dwarfs are at the high-mass end of the strip. Above this strip at the high-mass end of the range are the stars. In the lower-right are the most compact objects: neutron stars and black holes.
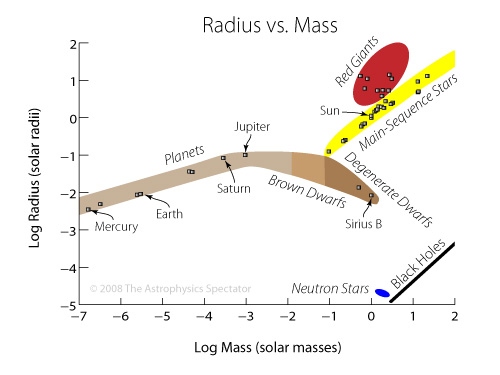
Radius versus mass of self-supporting objects. This diagram roughly shows how the sizes of various objects depend on their masses. The horizontal axis is the log base 10 of the mass in units of solar mass. The vertical axis is the log base 10 of the radius in units of solar radius. The squares mark specific objects with measured radii and masses. The planets, brown dwarfs, and degenerate (white) dwarfs lie on a continuous band extending from the smallest masses to 1.4 solar masses. The light brown region of this band encloses the planets, the medium brown region encloses the brown dwarfs, and the dark brown region encloses the degenerate dwarfs. The main-sequence stars lie in a yellow band extending from 0.072 solar masses to several hundred solar masses. The red giants are in the red region of the diagram. The neutron stars, which range in mass from about 1.4 to 2.5 solar masses, lie in the blue region at the bottom-right of the diagram, and the black holes lie along the black diagonal line at the bottom-right. The masses and radii for the two degenerate dwarfs in the plot (40 Eridani B and Sirius B) are from Shipman et al. (1997) and Provencal et al. (1998).[1,2] The masses and radii for the main-sequence stars and red giants are from Pasinetti Fracassini et al. (2001), Perevozkina and Svechnikov (1999), and Belikov (1995).[3,4,5]
The least-massive planets, which are composed of atoms, have densities that are nearly independent of mass, because the size of an atom inside the body does not change appreciably as the pressure increases. As a consequence, the mass of a small planet is directly proportional to the volume, so the radius of a small planet is proportional to the cube root of the mass. The four inner plants of the Solar System show this effect. Mars, which is 0.11 times Earth's mass, has a radius that is 0.53 times Earth, which is very close to the expected ratio of 0.48. The small deviation from the expected relationship between radius and mass occurs because the chemical composition of the planets differ and because there is a slight increase in density with an increase in the internal pressure.
When a planet becomes very massive?as massive as Saturn?something interesting happens: the high pressure frees the electrons from the atomic nuclei. If the interior temperature of the planet is low enough, these electrons provide all of the pressure. The electrons are in what is called a degenerate state. The pressure exerted by degenerate electrons depends only on the density of the electrons; temperature plays no role. This is the state that planets like Saturn and Jupiter, brown dwarfs, and degenerate dwarfs (white dwarfs) find themselves.
The material inside a degenerate object like Saturn is softer than in the smaller planets; unlike the solid rock of Earth, the material at the center of Saturn gives when it is squeezed. This means that as the mass of a degenerate object increases, which increases the pressure required to counter the object's self-gravity, the density also increases. The consequence is that the radius can decrease as the mass increases. For cold bodies of the same composition, the radius goes as the inverse of the cube root of the mass. For bodies with some internal heat?and generally there is some internal heat left over from the creation of the body?the radius decreases more slowly than for the cold bodies as the mass rises. This residual heat causes Jupiter to be slightly larger than Saturn, and it causes most of the known brown dwarfs to be about the size of, rather than much smaller than, Jupiter. The trend of smaller radius with larger mass extends up to the degenerate dwarfs, which are about the size of Earth. The ratio of Sirius B's (the white dwarf in the Sirius binary system) radius to Saturn's radius is the ratio of their masses to the ?0.28 power, which is reasonably close to the ?1/3 power expected from simple arguments that ignore differences in composition and the structure of the outer, non-degenerate regions of each body. The giant gaseous planets are therefore fundamentally linked to the degenerate dwarfs through electron degeneracy.
Very massive degenerate dwarfs do not exist. The reason is that electron degeneracy pressure cannot counteract gravity when the mass exceeds 1.4 solar masses. Objects with masses between about 1.4 and 2.5 solar masses are supported by the degeneracy pressure of protons and neutrons. These compact objects, which are the neutrons stars, are as dense as atomic nuclei. Above about 2.5 solar masses, nothing can stabilize the object, and, under the theory of general relativity, it becomes a black hole?an object that appears to us as a pure gravitational field.
The planets, the brown dwarfs, the degenerate dwarfs, the neutron stars, and the black holes are all permanent. They are the endpoints of evolution. The evolution after birth of the least-massive bodies is rapid enough that we only see these endpoints, but the evolution of the more massive objects, those with more than 0.072 solar masses, is slowed by the release of thermonuclear energy, so that we see most of them far from their final states. These are the stars. In a star, the pressure is not provided by cold electrons, but by hot electrons, ions, and light. Thermonuclear fusion of hydrogen provides energy to a main-sequence star at a rate that counters the loss of energy into space. This equilibrium fixes the temperature at the star's center. The heavier stars have a slightly higher internal temperature than the lighter stars. Because hydrogen fusion sets a star's core temperature, it also sets the core pressure. The consequence is that the thermonuclear fusion sets the relationship between a star's radius and mass. Taking the internal temperature to be a constant, one expects the radius of the star to be nearly proportional to the mass. For a red giant, the thermonuclear fusion is more complex, occurring at a variety of locations within the star. For this reason, the relationship between mass and radius in a red giant is not so simple.
Of objects that support themselves against gravitational collapse for very long times, the stars are the largest. The stars eventually do become smaller, becoming degenerate dwarfs. Stars like the Sun become degenerate dwarfs after about 9 billion years after their birth. The least massive stars, however, require hundreds of billions of years to become degenerate dwarfs, and because the universe is no more than 16 billion years old, no low-mass star has yet reached this final state.
[1]Shipman, H.L., Provencal, J.L., Hg, Erik, and Thejll, P. ?The Mass and Radius of 40 Eridani B from Hipparcos: An Accurate Test of Stellar Interior Theory.? The Astrophysical Journal Letters 488 (10 October 1997): L43?46.
[2]Provencal, J.L., Shipman, H.L., Hg, Erik, and Thejll, P. ?Testing the White Dwarf Mass-Radius Relation with Hipparcos.? The Astrophysical Journal 494 ( 20 February 1998): 759?767.
[3]Pasinetti Fracassini, L. E., Pastori, L, Covino, S., and Pozzi, A. ?Catalogue of Apparent Diameters and Absolute Radii of Stars (CADARS)?Third Edition?Comments and Statistics.? Astronomy and Astrophysics 367 (2001): 521?524. Available through the VizieR Service as catalog II/224.
[4]Perevozkina E.L., and Svechnikov M.A. ?Catalog of Eclipsing Binaries Parameters.? (1999). Available through the VizieR Service as catalog V/118.
[5]Belikov, A.N. ?Stellar Mass Catalogue.? Available through the VizieR Service as catalog V/85A.
